Making Sense of Physics Sensemaking

Elizabeth Gire, Oregon State University
Paul J. Emigh, Oregon State University
Kelby T. Hahn, Oregon State University
MacKenzie Lenz, Oregon State University

Physics instruction ought to reflect both the nature of physics and what we know about how people learn. Einstein described science as “the attempt to make the chaotic diversity of our sense-experience correspond to a logically uniform system of thought.”¹ This definition highlights how science is about making connections, both between what we experience every day and how we describe the world, as well as between the various logical connections within our descriptions.

Our models of the world use a variety of representations of knowledge, including equations, graphs, diagrams, conceptual stories, and experiences, encompassing both real-world experiences and experimental observations. Our colleague Dr. Charles de Leone often says that “Physicists are representation junkies.”² He is right—we try to use every tool at our disposal to make sense of the universe, always searching for new insights. Combining these perspectives, we might view physics sensemaking as seeking coherence between different representations of physics knowledge.

Solution Evaluation

Physics sensemaking may occur at many different stages of a physics problem: when initially identifying a problem, when orienting to a new problem, when stuck on a problem before a solution is reached, or when a solution is reached and you want to build confidence in your answer. Here, we focus on this last aspect of physics sensemaking: solution evaluation. By this, we mean examining an algebraic answer to a physics problem and probing whether it is reasonable. Students often have the luxury of being able to check against a solution manual or having their solution evaluated by a teacher. Research physicists have no solution manual: all we can do is make sure our solutions are consistent with what we already know and that they make predictions about the behavior of the universe that can be verified. Students must develop the skills and habits to evaluate their own solutions. Here we discuss two big categories of answer evaluation: examining “beasts” and answer contextualization/comparison (Figure 1).

 

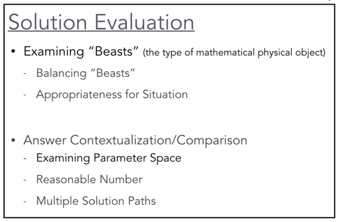
Figure 1: Types of Solution Evaluation

What kind of a beast is it?
When we arrive at an answer to a physics problem, often we want to make sure it is the right type of mathematical and physical object. In classes at Oregon State, we ask students “What kind of a beast is it?” meaning “what kind of a mathematical/physical object is it?” We ask this question for two reasons. First, we want to make sure the answer is appropriate for the problem that is being solved. If you are solving for an angular momentum, you better have a vector with dimensions of angular momentum. Second, if we have an equation (which we usually do), we want to make sure that the equation is balanced: if you have a vector with dimensions of angular momentum on one side of an equals sign, you should have the same dimensions on the other side (similarly, you want every term in an equation to be consistent).

Answer Contextualization/Comparison

A second way to evaluate an answer is to try to understand the answer in context or compare the answer to something you already know. Strategies for this include examining parameter space (including checking special or limiting cases and examining the relationships between variables—see Figure 2), telling a conceptual story, checking against observations/intuition, and making sure a numerical answer has a reasonable magnitude.

 

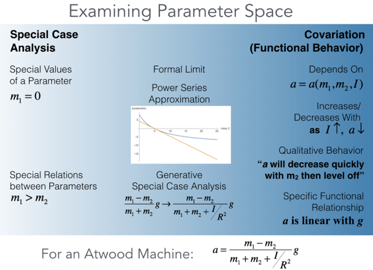
Figure 2: The spectrum of strategies that includes exploring the parameter space of an algebraic solution illustrated through the example of the acceleration of an Atwood machine with a massive pulley.

A Course in Physics Sensemaking

Recently, Oregon State University did a major revision of the physics major, including the addition of a course called Techniques in Theoretical Mechanics. This course is one of two new sophomore-level courses aimed at (1) easing the transition between introductory and upper-division physics courses and (2) bringing more of the “cool” physics earlier in the major to interest new (and perhaps underrepresented) populations of students.

In this mechanics course, physics sensemaking is on equal footing with the physics and math content of the course. Evaluative sensemaking strategies are discussed and practiced in class, on homework, and on exams. The course is generally modeled after a course on mathematical problem solving offered by Alan Schoenfeld at UC Berkeley in the 1980’s.³ The design of the physics sensemaking course attends to four aspects of physics sensemaking: knowledge of sensemaking strategies (and physics content), metacognitive skills, productive beliefs about the nature of doing physics, and valuing physics sensemaking. Sensemaking strategies are named and described in class and on course assignments. Instructors use Schoenfeld’s metacognitive prompts (What are you doing? Why are you doing it? How will it help you?) to support routine self-monitoring.⁴ The course is pitched as professional development for future physicists; physics epistemology and professional sensemaking practice is discussed. Demonstrations of physics sensemaking are rewarded with grades on course assignments and praise in class discussions.

In terms of physics content (one cannot do physics sensemaking in the absence of physics!), the course begins with using Newton’s Laws to find equations of motion in situations where forces depend on velocity. Students learn to view Newton’s 2nd law as a differential equation of motion and to solve separable differential equations to find velocities and positions as functions of time. Students are introduced to hyperbolic trig functions in the context of quadratic drag forces, which become useful later in doing Lorentz transformations in special relativity. Students then learn Lagrangian and Hamiltonian approaches to finding and solving equations of motion for classical systems.⁵ Students learn to leverage their intuitions of classical systems to make sense of messy algebraic calculations. The course ends with special relativity taught with an emphasis on using spacetime diagrams as a bridge between conceptual, geometric, and algebraic modes of reasoning.⁶ Here, students learn to use physics sensemaking to develop and refine their intuitions about relativistic physics.

The hope is that doing physics sensemaking becomes a habitual part of solving physics problems for the students. To support this goal, we use a scaffolding and fading approach⁷ in which students are given explicit support at the beginning of the course that is gradually removed as the course proceeds. Students initially receive instructions for how and when to use specific physics sensemaking strategies. Sensemaking strategies are tagged on homework assignments in appropriate places. At the beginning of the term, in the context of solving a projectile motion problem, the class generates a list of physics sensemaking strategies that is made available as a resource for the remainder of the course. On later homework assignments, sensemaking prompts become less prescribed: “Use at least 3 strategies to make sense of your answer.” Eventually, sensemaking is mentioned as an expectation in the assignment instruction but not specifically prompted in any problem.

Preliminary Results

We are still in the early days of this project but we have some preliminary results to report. At the beginning of this course, students are familiar with many different strategies for making sense of physics problems,⁸ but often the implementation of these strategies needs support.⁹ For example, in examining a special case of an algebraic solution, most students can evaluate functions at special values and interpret the result, but some need to learn how to select a good special case to examine (from physical intuition or from a known result).¹⁰ Students may also not recognize a strategy that they can perform as being useful for physics sensemaking. For example, one of our students did not recognize examining the functional relationships between physical variables with a graph as a way of making sense of an answer to a physics problem.¹¹ Overall, a variety of data sources—including pre/post-test data, in-class observations, analysis of homework and exams, interviews with individual students, an end-of-course survey, and anecdotal reports from our faculty colleagues—students generally become more familiar, more proficient, and more productive with strategies for making sense of symbolic answers to physics problems. Our next steps include following up with students to see how their experiences in the course have influenced their physics sensemaking practices in later courses and research experiences, characterizing and developing curriculum for physics sensemaking beyond solution evaluation, and developing assessments of physics sensemaking.

Elizabeth Gire is an assistant professor of physics at Oregon State University. She conducts research on the teaching and learning of physics, particularly physics sensemaking and representational fluency.

Paul J. Emigh is a Postdoctoral Scholar at Oregon State University. His research focuses on how students at all levels of physics understand and make sense of both physics concepts and the underlying mathematics.

Kelby T. Hahn is a doctoral student in STEM Education at Oregon State University. She works in Dr. Gire’s physics education research group studying physics sensemaking, specifically special-case analysis.

MacKenzie Lenz is a doctoral candidate in Physics at Oregon State University. She works in the Oregon State University Physics Education Research group studying student performance of and beliefs surrounding sensemaking at different levels of the undergraduate physics curriculum.

(Endnotes)

1. Albert Einstein, “Considerations concerning the fundaments of theoretical physics.” Science, 91(2369), 487–492 (1940).

2. Charles De Leone, private communication.

3. Alan H. Schoenfeld, “Chapter 1 - A framework for the analysis of mathematical behavior,” in Mathematical Problem Solving Academic Press, pp. 11–45 (1985).

4. Alan H. Schoenfeld, “Chapter 4 - Control,” in Mathematical Problem Solving, Academic Press, pp. 11–45 (1985).

5. John R. Taylor, Classical Mechanics, University Science Books (2005).

6. Tevian Dray, The Geometry of Special Relativity Boca Raton, FL: A K Peters/CRC Press, (2012).

7. Barak Rosenshine and Carla Meister, “The use of scaffolds for teaching higher-level cognitive strategies” Educational Leadership, 49(7), 26–33 (1992).

8. Kelby T. Hahn, Paul J. Emigh, MacKenzie Lenz, and Elizabeth Gire, “Student Sense-making on Homework in a Sophomore Mechanics Course,” 2017 PERC Proceedings [Cincinnati, OH, July 26-27], 160-163 (2017).

9. MacKenzie Lenz, Kelby T. Hahn, Paul J. Emigh, and Elizabeth Gire, “Student perspective of and experience with sense-making: a case study,” 2017 PERC Proceedings [Cincinnati, OH, July 26-27], 240-243 (2017).

10. Kelby T. Hahn. “Student evaluative sensemaking on homework in intermediate mechanics,” Master’s Thesis, Oregon State University, (2018).

11. MacKenzie Lenz, Paul J. Emigh, and Elizabeth Gire, “Surprise! Students don’t do special-case analysis when unaware of it,” 2018 PERC Proceedings [Washington, DC, August 1-2], (accepted).

---

Disclaimer – The articles and opinion pieces found in this issue of the APS Forum on Education Newsletter are not peer refereed and represent solely the views of the authors and not necessarily the views of the APS.